A line segment is a part of a line that is bounded by two distinct endpoints, containing every point on the line between its endpoints, and at WHAT.EDU.VN, we make understanding geometry easy and free. This defined length makes it a fundamental concept in geometry, distinct from lines and rays, playing a crucial role in shapes, measurements, and spatial understanding. Explore the properties of line segments including length, midpoint, congruence, and their practical applications in real-world scenarios for deeper insights into geometric principles, and learn more with us about geometric shapes, geometrical figures and geometrical concepts.
1. What is a Line Segment?
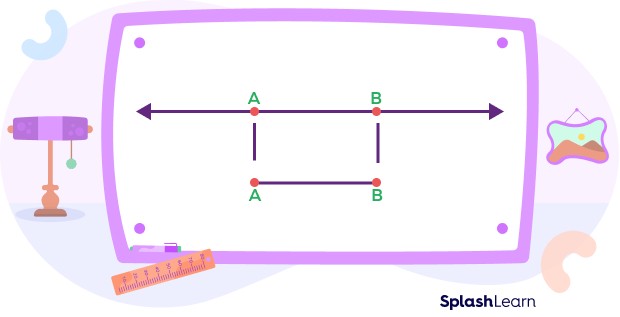
A line segment is a section of a straight line that is defined by two endpoints and includes all the points between them. Unlike a line, which extends infinitely in both directions, a line segment has a specific, measurable length.
- Definition: A part of a line with two endpoints.
- Characteristics: Definite length, measurable.
- Symbol: Represented by a bar over the letters of the endpoints (e.g., $overline{AB}$).
2. What is the Difference Between a Line, a Ray, and a Line Segment?
Understanding the differences between a line, a ray, and a line segment is essential in geometry. Each has unique properties:
- Line: Extends infinitely in both directions, has no endpoints.
- Ray: Has one endpoint and extends infinitely in one direction.
- Line Segment: Has two endpoints and a definite length.
| Feature | Line | Ray | Line Segment |
|---|---|---|---|
| Endpoints | None | One | Two |
| Length | Infinite | Infinite | Definite, measurable |
| Representation | $overleftrightarrow{AB}$ | $overrightarrow{AB}$ | $overline{AB}$ |
| Example | A perfectly straight, endless road | A laser beam | The edge of a table |
3. How Do You Measure the Length of a Line Segment?
Measuring the length of a line segment is a fundamental skill in geometry. Here’s a step-by-step guide:
- Tools: Use a ruler or measuring tape.
- Placement: Align the zero mark of the ruler with one endpoint of the line segment.
- Reading: Read the value on the ruler where the other endpoint falls.
- Units: Record the length along with the appropriate unit (e.g., cm, inches).
4. What are the Units Used to Measure a Line Segment?
Line segments can be measured using various units, depending on the context and the scale of the segment:
- Metric Units:
- Millimeters (mm): For very small lengths.
- Centimeters (cm): Commonly used for everyday measurements.
- Meters (m): For larger lengths.
- Kilometers (km): For very long distances.
- Imperial Units:
- Inches (in): Commonly used in the United States.
- Feet (ft): Used for measuring larger lengths.
- Yards (yd): Used in construction and sports.
- Miles (mi): For long distances, like measuring roads.
5. How Can You Draw a Line Segment of a Specific Length?
Drawing a line segment of a specific length requires precision. Here’s how to do it using a ruler and a pencil:
- Preparation: Have a ruler, a pencil, and a piece of paper.
- Starting Point: Mark a point on the paper; this will be one endpoint.
- Alignment: Place the ruler’s zero mark on the starting point.
- Endpoint: Find the desired length on the ruler and mark another point.
- Connect: Draw a straight line connecting the two points using the ruler as a guide.
6. What is the Midpoint of a Line Segment?
The midpoint of a line segment is the point that divides the segment into two equal parts.
- Definition: The point exactly halfway between the two endpoints.
- Calculation: If the endpoints are $(x_1, y_1)$ and $(x_2, y_2)$, the midpoint is $left(frac{x_1 + x_2}{2}, frac{y_1 + y_2}{2}right)$.
- Importance: Useful in geometry for bisecting segments and constructing shapes.
7. What is Line Segment Congruence?
Line segment congruence refers to the equality of two or more line segments in terms of their lengths.
- Definition: Two line segments are congruent if they have the same length.
- Symbol: Congruence is denoted by the symbol $cong$. So, if segment $overline{AB}$ is congruent to segment $overline{CD}$, we write $overline{AB} cong overline{CD}$.
- Significance: Congruence is a fundamental concept in geometry, used to prove properties of shapes and figures.
8. What are Real-Life Examples of Line Segments?
Line segments are found everywhere in the real world. Recognizing them helps in understanding geometric concepts better:
- Edges of Objects: The edges of tables, books, and picture frames.
- Structural Components: Sides of buildings, fences, and bridges.
- Everyday Items: Pencils, rulers, and matchsticks.
- Digital Displays: The individual lines that make up characters on a digital screen.
9. How are Line Segments Used in Geometry?
Line segments are foundational to many concepts in geometry:
- Shapes: Forming the sides of polygons such as triangles, squares, and rectangles.
- Constructions: Used in geometric constructions with compasses and rulers.
- Proofs: Essential in proving geometric theorems and properties.
- Coordinate Geometry: Defining paths and distances on coordinate planes.
10. What are Parallel Line Segments?
Parallel line segments are segments that lie on parallel lines and never intersect, no matter how far they are extended.
- Definition: Line segments that are equidistant from each other and do not intersect.
- Characteristics: Have the same slope if they are on a coordinate plane.
- Examples: Opposite sides of a rectangle or square.
11. What are Perpendicular Line Segments?
Perpendicular line segments intersect at a right angle (90 degrees).
- Definition: Line segments that meet at a 90-degree angle.
- Characteristics: Their slopes are negative reciprocals of each other if they are on a coordinate plane.
- Examples: The intersection of the height and base of a right triangle.
12. How Do You Calculate the Distance Between Two Points Using a Line Segment?
The distance between two points in a coordinate plane can be found using the distance formula, which is derived from the Pythagorean theorem. This distance represents the length of the line segment connecting the two points.
-
Formula: If the points are $(x_1, y_1)$ and $(x_2, y_2)$, the distance $d$ is given by:
$d = sqrt{(x_2 – x_1)^2 + (y_2 – y_1)^2}$
-
Application: This formula is used in various fields, including navigation, engineering, and computer graphics.
13. What is a Bisector of a Line Segment?
A bisector of a line segment is a line, ray, or segment that passes through the midpoint of the line segment, dividing it into two congruent segments.
- Definition: A line that cuts a line segment into two equal parts at its midpoint.
- Types: Can be a line, ray, or another line segment.
- Importance: Used in geometric constructions and proofs.
14. How Do Line Segments Relate to Triangles?
Line segments form the sides of triangles, and understanding their properties is crucial for analyzing triangles:
- Triangle Inequality Theorem: The sum of the lengths of any two sides of a triangle must be greater than the length of the third side.
- Types of Triangles: Triangles are classified based on the lengths of their sides (e.g., equilateral, isosceles, scalene).
- Medians: A median of a triangle is a line segment from a vertex to the midpoint of the opposite side.
15. What Role Do Line Segments Play in Polygons?
Polygons are closed figures made up of line segments. These segments form the sides of the polygon, and their properties determine the characteristics of the polygon:
- Definition: A closed, two-dimensional shape formed by line segments.
- Examples: Squares, rectangles, pentagons, and hexagons.
- Properties: The lengths and angles of the line segments determine the shape and size of the polygon.
16. How Do Line Segments Apply to Coordinate Geometry?
In coordinate geometry, line segments are used to define and analyze geometric figures on a coordinate plane:
- Endpoints: Each line segment is defined by the coordinates of its endpoints.
- Slope: The slope of a line segment indicates its direction and steepness.
- Equations: Line segments can be represented by linear equations.
17. What are the Applications of Line Segments in Engineering?
Line segments are fundamental in engineering design and construction:
- Structural Design: Used to represent beams, columns, and other structural elements.
- CAD Software: Utilized in computer-aided design (CAD) software to create detailed models.
- Surveying: Used to measure distances and create maps.
18. How are Line Segments Used in Computer Graphics?
In computer graphics, line segments are essential for creating images and animations:
- Vector Graphics: Images are created using lines and curves defined by mathematical equations.
- 3D Modeling: Used to construct the edges of 3D objects.
- Animation: Motion is created by manipulating the positions and properties of line segments.
19. What is the Difference Between a Chord and a Line Segment in a Circle?
In the context of circles, both chords and line segments are important, but they have distinct properties:
- Chord: A line segment whose endpoints both lie on the circle.
- Diameter: A special chord that passes through the center of the circle.
- Tangent: A line that touches the circle at only one point; it is not a line segment but related to the geometry of circles.
20. How Do You Divide a Line Segment into Equal Parts?
Dividing a line segment into equal parts is a common geometric construction. Here’s how to do it using a compass and straightedge:
- Draw a Line: Start with the line segment you want to divide.
- Create an Angle: Draw a line at an arbitrary angle from one endpoint.
- Mark Equal Segments: Use a compass to mark equal segments along the new line.
- Connect the Last Point: Connect the last point to the other endpoint of the original segment.
- Draw Parallel Lines: Draw lines parallel to the connecting line from each mark on the angled line. These will divide the original segment equally.
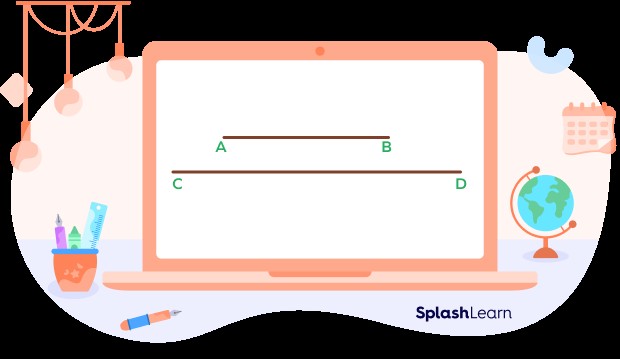
21. What are Collinear Line Segments?
Collinear line segments are segments that lie on the same straight line.
- Definition: Segments that are part of the same line.
- Characteristics: Their endpoints lie on a single line.
- Importance: Used in geometry to prove properties of lines and figures.
22. How Do You Find the Equation of a Line Segment in Coordinate Geometry?
Finding the equation of a line segment involves determining the linear equation that represents the line on which the segment lies:
- Find the Slope: Use the formula $m = frac{y_2 – y_1}{x_2 – x_1}$ with the coordinates of the endpoints.
- Use Point-Slope Form: $y – y_1 = m(x – x_1)$, then convert to slope-intercept form ($y = mx + b$) or standard form ($Ax + By = C$).
- Define the Segment: Specify the range of $x$ or $y$ values that define the line segment between its endpoints.
23. What is the Golden Ratio and How Does it Relate to Line Segments?
The golden ratio, approximately 1.618, is a mathematical constant that appears in various areas of mathematics and art. It relates to line segments when a segment is divided into two parts such that the ratio of the whole segment to the longer part is the same as the ratio of the longer part to the shorter part.
- Definition: An irrational number often denoted by the Greek letter phi ($phi$).
- Application: Used in art and architecture to create aesthetically pleasing proportions.
- Formula: If a line segment of length $a + b$ is divided into lengths $a$ and $b$, then $frac{a+b}{a} = frac{a}{b} = phi$.
24. How Do Line Segments Contribute to Understanding Perimeter and Area?
Line segments are essential for calculating the perimeter and area of various shapes:
- Perimeter: The sum of the lengths of the line segments that form the boundary of a shape.
- Area: The amount of space enclosed within the line segments of a two-dimensional shape.
- Formulas: Different shapes have different formulas for calculating perimeter and area based on the lengths of their line segments.
25. What are the Key Properties of Line Segments in Geometric Proofs?
Line segments have several key properties that are used in geometric proofs:
- Definite Length: They have a measurable length.
- Midpoint: They have a midpoint that divides them into two congruent segments.
- Congruence: Two segments are congruent if they have the same length.
- Collinearity: Segments can be collinear if they lie on the same line.
26. How Do You Construct a Perpendicular Bisector of a Line Segment?
Constructing a perpendicular bisector is a common geometric task:
- Draw Arcs: Place the compass at one endpoint and draw an arc that extends more than halfway across the segment.
- Repeat: Repeat from the other endpoint, ensuring the compass width is the same.
- Draw the Bisector: Draw a line through the points where the arcs intersect. This line is the perpendicular bisector.
27. What is the Importance of Line Segments in Mapmaking?
Line segments are crucial in mapmaking for representing distances and boundaries:
- Scale: Maps use a scale to represent real-world distances with line segments on the map.
- Boundaries: Line segments define the borders of countries, states, and cities.
- Routes: Roads and paths are represented by line segments on maps.
28. How Do You Use Line Segments to Create Tessellations?
Tessellations are patterns made of repeating shapes that cover a plane without gaps or overlaps. Line segments form the sides of these shapes:
- Regular Tessellations: Made of congruent regular polygons (e.g., equilateral triangles, squares, hexagons).
- Semi-Regular Tessellations: Made of two or more different regular polygons.
- Creating Tessellations: Line segments must fit together perfectly at vertices to avoid gaps or overlaps.
29. What are the Different Types of Line Segment Relationships in Geometry?
Line segments can have various relationships with each other in geometric figures:
- Adjacent: Sharing a common endpoint.
- Intersecting: Crossing each other at a point.
- Parallel: Never intersecting and equidistant.
- Perpendicular: Intersecting at a right angle.
- Congruent: Having the same length.
30. How Can You Use Line Segments to Teach Basic Geometry Concepts?
Line segments are an excellent tool for teaching basic geometry concepts to students:
- Visual Aid: Easy to draw and manipulate, making them great for visual learners.
- Hands-On Activities: Can be used in activities involving measurement, construction, and pattern recognition.
- Foundation: Provide a solid foundation for understanding more complex geometric concepts.
Do you have any questions or need further clarification on line segments? Visit WHAT.EDU.VN and ask your question for free! Our community of experts is ready to provide you with clear, helpful answers.
Line segments are fundamental building blocks in geometry, with applications spanning various fields from engineering to computer graphics. Understanding their properties and relationships is essential for mastering geometric concepts.
Ready to learn more and get your questions answered? Head over to WHAT.EDU.VN now. Whether you need help with homework, understanding a concept, or exploring new topics, our platform is here to assist you. Don’t struggle alone – ask your question today and get the answers you need for free.
For any inquiries, you can reach us at 888 Question City Plaza, Seattle, WA 98101, United States, or via WhatsApp at +1 (206) 555-7890. Visit our website at what.edu.vn to get started. We’re here to help you succeed!