What Is An Angle? WHAT.EDU.VN provides a comprehensive explanation of what an angle is, exploring its definition, various types, and real-world applications. Discover how this fundamental geometric concept is used daily and find out how you can get your angle questions answered quickly and easily on WHAT.EDU.VN. Explore angle measurement, angle types, and geometric shapes.
1. Angle: The Meeting Point of Lines and Rays
An angle is formed when two straight lines or rays meet at a common endpoint. This common point is known as the vertex. The term “angle” originates from the Latin word “angulus,” meaning “corner,” perfectly capturing the essence of what an angle represents. Understanding angles is fundamental to geometry and essential for various applications in math, science, and everyday life.
2. The Angle Symbol and Measurement
The symbol ∠ is used to represent an angle. Angles are measured in degrees (°), a unit that quantifies the amount of rotation. A protractor is the tool used to measure angles accurately. For example, an angle of 45 degrees is written as 45°.
3. Parts of an Angle Explained
An angle comprises several key components:
- Vertex: The vertex is the point where the two lines or sides of the angle meet. In mathematical diagrams, the vertex is often labeled with a letter, such as “O.”
- Arms: The two sides of the angle that extend from the vertex are referred to as arms. These arms define the angle’s opening or spread.
- Initial Side: Also called the reference line, the initial side is the starting point from which the angle is drawn. It is a straight line that serves as the base for measuring the angle.
- Terminal Side: The terminal side is the line that indicates the end of the angle’s measurement. It is the side up to which the angle is measured from the initial side.
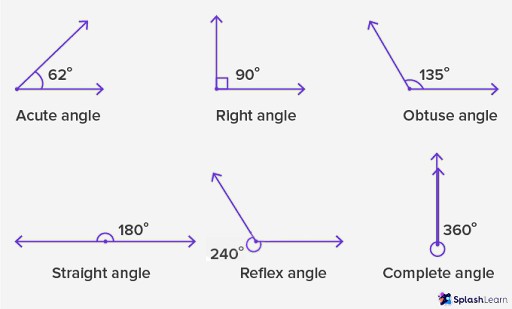
4. Discover the Types of Angles
Angles are classified into different types based on their measurements:
- Acute Angle: An acute angle measures less than 90°. It is a small, sharp angle.
- Right Angle: A right angle measures exactly 90°. It is often represented by a small square at the vertex.
- Obtuse Angle: An obtuse angle measures greater than 90° but less than 180°. It is a wide, blunt angle.
- Straight Angle: A straight angle measures exactly 180°. It forms a straight line.
- Reflex Angle: A reflex angle measures greater than 180° but less than 360°. It is a large angle that “reflexes” back on itself.
- Complete Angle: A complete angle measures exactly 360°. It forms a full circle.
5. Interior and Exterior Angles Explained
Angles can also be classified based on their position relative to a shape:
- Interior Angles: Interior angles are the angles formed inside a shape. The sum of the interior angles of a triangle, for example, is always 180°.
- Exterior Angles: Exterior angles are the angles formed outside a shape, between one of its sides and an extension of an adjacent side.
6. Complementary and Supplementary Angles Defined
- Complementary Angles: Two angles are complementary if their measures add up to 90°.
- Supplementary Angles: Two angles are supplementary if their measures add up to 180°.
7. How to Construct an Angle with a Protractor
To construct an angle using a protractor, follow these steps:
- Draw a ray OA of any length. This will be the initial side of your angle.
- Place the protractor so that its midpoint is on point O, the vertex of the angle.
- Mark the desired angle on the protractor’s circular scale. For example, if you want to construct a 40° angle, mark the point at 40°.
- Draw a straight line from point O through the marked point on the protractor. This will be the terminal side of your angle.
- Indicate the degree of the angle where the two sides intersect.
8. Real-World Applications of Angles
Angles are used in numerous real-world applications:
- Engineering: Engineers use angles to design and construct buildings, bridges, and other structures.
- Sports: Athletes use angles to enhance their performance in sports such as golf, basketball, and soccer.
- Carpentry: Carpenters use angles to make furniture, doors, and other wooden structures.
- Art: Artists use angles to create perspective and depth in their drawings and paintings.
- Timekeeping: Clocks use angles to display the time with hour and minute hands.
9. Everyday Examples of Angles
Angles are all around us in everyday life:
- Clothes hangers
- Arrowheads
- Scissors
- Partly opened doors
- Pyramids
- Edges of tables
- Edges of rulers
- Pizza Slices
10. Solved Examples: Putting Angle Concepts into Practice
Let’s explore some solved examples to illustrate how angle concepts are applied in problem-solving.
Example 1: Finding a Missing Angle
Find the missing angle x in the figure, given that ∠x + 35° = 90°.
Solution:
To find the missing angle x, we can subtract 35° from 90°:
∠x = (90 – 35)° = 55°
Example 2: Solving for x Using Alternate Angles
Solve for x, given that 5x – 70 = 105 (alternate angles).
Solution:
To solve for x, we first add 70 to both sides of the equation:
5x = 175
Then, we divide both sides by 5:
x = 35°
Example 3: Finding an Angle in a Triangle
In a triangle ABC, ∠A = 90° and ∠B = 30°. Find ∠C.
Solution:
The sum of all three interior angles of a triangle is equal to 180°. Therefore, we can write the equation:
90° + 30° + x = 180°
Solving for x:
x = 180 – (90 + 30) = 60°
Therefore, ∠C = 60°.
11. Practice Problems: Test Your Angle Knowledge
Test your understanding of angles with these practice problems.
Problem 1: The sum of all angles around a point equals:
a) 90°
b) 180°
c) 270°
d) 360°
Answer: d) 360°
Problem 2: Angles that sum up to 90° are known as:
a) Vertical angles
b) Complementary angles
c) Reflexive angles
d) Supplementary angles
Answer: b) Complementary angles
Problem 3: Angles that are opposite to each other when two straight lines intersect are called:
a) Vertical angles
b) Complementary angles
c) Reflexive angles
d) Supplementary angles
Answer: a) Vertical angles
12. Frequently Asked Questions About Angles
Let’s address some frequently asked questions about angles to clarify any remaining doubts.
12.1 What are the types of angles based on the direction of a cycle?
Angles can be classified based on their direction of measurement:
- Positive Angles: Measured in the counterclockwise direction from the baseline.
- Negative Angles: Measured in the clockwise direction from the baseline.
12.2 Do angles on a straight line always add up to 180°?
Yes, angles that share a vertex and one side of a line always add up to 180°. These are called supplementary angles.
12.3 What are the types of angles based on measurement?
Based on measurement, angles are classified as:
- Acute angle
- Right angle
- Obtuse angle
- Straight angle
- Reflex angle
- Complete angle
12.4 What is the application of angles in math?
Angles are used in various mathematical applications, including:
- Geometry
- Trigonometry
- Calculus
- Engineering
- Architecture
Engineers and architects use angles for building, measurement, and designing structures.
13. Understanding Angles in Navigation
Angles play a crucial role in navigation, helping determine direction and location. Whether it’s sailing the seas or flying across continents, angles guide us on our journeys.
14. How Angles Impact Computer Graphics
In computer graphics, angles are fundamental to creating realistic and visually appealing images. From rotating objects to simulating light and shadows, angles are at the heart of digital artistry.
15. Angles in Music: Understanding Harmony
Even in music, angles can be used to understand harmony and rhythm. The relationships between musical notes can be represented using angles, providing a visual way to understand musical composition.
16. The Role of Angles in Astronomy
Astronomy relies heavily on angles to measure the positions of stars, planets, and other celestial objects. These angular measurements help astronomers map the universe and understand its structure.
17. Angles and the Human Body
Our bodies are full of angles, from the joints in our limbs to the angle of our spine. Understanding these angles is crucial for biomechanics and physical therapy.
18. Creating Art with Angles: Geometric Design
Geometric design uses angles to create visually stunning patterns and artwork. This form of art relies on precise measurements and relationships between angles to achieve its aesthetic appeal.
19. Angles in Robotics: Precision and Control
In robotics, angles are essential for controlling the movement and precision of robotic arms and other devices. Accurate angular measurements ensure that robots can perform their tasks with accuracy and efficiency.
20. Using Angles to Understand Fractals
Fractals are complex geometric shapes that exhibit self-similarity at different scales. Angles play a key role in defining and understanding these intricate patterns.
21. The Importance of Angles in Construction
Construction projects rely heavily on angles to ensure that structures are stable and meet safety standards. From the foundation to the roof, angles are crucial for every aspect of building design and construction.
22. Understanding the Golden Angle in Nature
The golden angle, approximately 137.5 degrees, appears in many natural phenomena, such as the arrangement of leaves on a stem and the spiral patterns of sunflowers. This angle optimizes space and light exposure, showcasing the mathematical beauty of nature.
23. Angles in Surveying: Mapping the Earth
Surveyors use angles to measure and map the Earth’s surface. These angular measurements are essential for creating accurate maps and defining property boundaries.
24. How Angles are Used in Video Games
Video games use angles to create realistic environments and character movements. Angles determine how objects interact with each other and how players perceive the game world.
25. Angles in Optical Illusions: Tricking the Eye
Optical illusions often exploit our perception of angles to create images that appear to be different from reality. These illusions demonstrate how our brains interpret angular information and create our visual experiences.
26. Exploring the World of Tessellations with Angles
Tessellations are patterns made up of repeating shapes that fit together without any gaps or overlaps. Angles play a crucial role in creating tessellations, ensuring that the shapes fit perfectly together.
27. The Significance of Angles in Aircraft Design
Aircraft design relies heavily on angles to optimize aerodynamics and ensure stable flight. The angles of the wings, tail, and other surfaces are carefully calculated to maximize lift and minimize drag.
28. Using Angles in Weather Forecasting
Weather forecasting uses angles to measure the direction and intensity of winds, sunlight, and other atmospheric phenomena. These angular measurements help meteorologists predict weather patterns and climate changes.
29. Angles in Photography: Capturing the Perfect Shot
Photographers use angles to frame their shots and create visually appealing compositions. The angle at which a photo is taken can dramatically affect the mood and impact of the image.
30. The World of Origami: Creating with Angles
Origami, the art of paper folding, relies heavily on angles to create intricate and beautiful designs. By precisely folding paper at specific angles, origami artists can transform a flat sheet into a three-dimensional sculpture.
Angles are indeed fundamental to understanding our world. Are you curious to learn more about geometric concepts or need help with a specific question? Visit WHAT.EDU.VN today and ask your question for free. Our community of experts is ready to provide you with the answers you need, quickly and accurately. Contact us at 888 Question City Plaza, Seattle, WA 98101, United States, or via Whatsapp at +1 (206) 555-7890. Explore the world of knowledge with what.edu.vn.