Are you struggling with understanding the distributive property? At WHAT.EDU.VN, we believe that everyone deserves access to clear, concise explanations. This article breaks down the distributive property with simple explanations, real-world examples, and practical tips to help you master this fundamental mathematical concept. Discover how this property can simplify complex calculations and improve your problem-solving skills.
1. Understanding the Distributive Property
The distributive property is a core principle in mathematics that connects multiplication with addition and subtraction. Essentially, it allows you to multiply a single term by two or more terms inside a set of parentheses. This property is incredibly useful for simplifying expressions and solving equations, turning seemingly complex problems into manageable steps. With the distributive property, you can approach any mathematical challenge with confidence.
The Distributive Law can be expressed as follows:
- A (B + C) = AB + AC
Where:
- A, B, and C represent real numbers.
- A is multiplied across both B and C.
1.1 The Essence of Distribution
To “distribute” means to divide or give out portions. In mathematical terms, the distributive property involves spreading multiplication over addition or subtraction within parentheses.
For example:
3 × (4 + 5) can be solved as (3 × 4) + (3 × 5).
1.2 Real-World Relevance
The distributive property isn’t just a theoretical concept; it has practical applications in everyday life. For instance, when calculating costs at a store or determining quantities in recipes, the distributive property can simplify your calculations.
Imagine you want to buy 3 notebooks priced at $2 each and 3 pens priced at $1 each. Instead of calculating each separately, you can use the distributive property:
3 × ($2 + $1) = (3 × $2) + (3 × $1) = $6 + $3 = $9
This not only simplifies the math but also shows how the distributive property works in a practical context.
2. Distributive Property Over Addition
The distributive property of multiplication over addition is applied when multiplying a number by the sum of two or more numbers.
2.1 Formula and Explanation
The formula for this property is:
- A (B + C) = AB + AC
This means multiplying ‘A’ by the sum of ‘B’ and ‘C’ is the same as multiplying ‘A’ by ‘B’ and ‘A’ by ‘C’ separately, and then adding the products.
2.2 Step-by-Step Examples
Let’s illustrate with an example:
Solve: 4 × (6 + 3)
-
Apply the Distributive Property:
- 4 × (6 + 3) = (4 × 6) + (4 × 3)
-
Perform the Multiplication:
- (4 × 6) = 24
- (4 × 3) = 12
-
Add the Products:
- 24 + 12 = 36
Therefore, 4 × (6 + 3) = 36
2.3 Practical Applications
Consider calculating the total cost of buying 5 apples and 5 bananas. If each apple costs $0.50 and each banana costs $0.30, you can use the distributive property:
5 × ($0.50 + $0.30) = (5 × $0.50) + (5 × $0.30) = $2.50 + $1.50 = $4.00
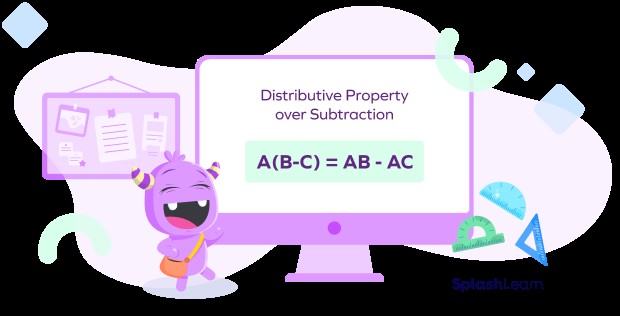
3. Distributive Property Over Subtraction
The distributive property of multiplication over subtraction is similar to that over addition but involves subtraction instead.
3.1 Formula and Explanation
The formula is:
- A (B – C) = AB – AC
This means multiplying ‘A’ by the difference of ‘B’ and ‘C’ is the same as multiplying ‘A’ by ‘B’ and ‘A’ by ‘C’ separately, and then subtracting the products.
3.2 Detailed Examples
Solve: 7 × (9 – 2)
-
Apply the Distributive Property:
- 7 × (9 – 2) = (7 × 9) – (7 × 2)
-
Perform the Multiplication:
- (7 × 9) = 63
- (7 × 2) = 14
-
Subtract the Products:
- 63 – 14 = 49
Therefore, 7 × (9 – 2) = 49
3.3 Everyday Scenarios
Imagine you are calculating the remaining money after buying items. You have $20, and you want to buy 3 items, each costing $6, but you have a coupon for $1 off each item.
3 × ($6 – $1) = (3 × $6) – (3 × $1) = $18 – $3 = $15
This shows the total amount you’ll spend after applying the discount.
4. Verification of the Distributive Property
To verify the distributive property, solve an expression using both the distributive property and the standard order of operations (PEMDAS/BODMAS).
4.1 Step-by-Step Verification
Example: Verify 5 × (8 + 4)
-
Using Distributive Property:
- 5 × (8 + 4) = (5 × 8) + (5 × 4)
- = 40 + 20
- = 60
-
Using Order of Operations:
- 5 × (8 + 4) = 5 × 12
- = 60
Both methods yield the same result, verifying the property.
4.2 Practical Checks
Consider a real-world check: You want to buy 4 sandwiches and 4 drinks. Each sandwich costs $5, and each drink costs $2. You can calculate the total cost in two ways:
-
Distributive Property:
- 4 × ($5 + $2) = (4 × $5) + (4 × $2)
- = $20 + $8
- = $28
-
Direct Calculation:
- 4 sandwiches at $5 each = $20
- 4 drinks at $2 each = $8
- Total = $20 + $8 = $28
Both calculations match, affirming the correctness of the distributive property.
5. How to Apply the Distributive Property
To effectively use the distributive property, follow these simple steps.
5.1 Distributing the Multiplier
Identify the multiplier (the term outside the parentheses) and distribute it to each term inside the parentheses.
Example: 6 × (7 + 2)
-
Distribute:
- 6 × 7 + 6 × 2
5.2 Performing Individual Multiplications
Multiply the multiplier by each term inside the parentheses.
-
Multiply:
- 6 × 7 = 42
- 6 × 2 = 12
5.3 Adding or Subtracting the Products
Add or subtract the results obtained from the individual multiplications.
-
Add:
- 42 + 12 = 54
Therefore, 6 × (7 + 2) = 54
5.4 Tips and Tricks
- Double-Check: Always double-check your multiplication to avoid errors.
- Sign Awareness: Pay attention to signs, especially when dealing with subtraction and negative numbers.
- Practice: Consistent practice will enhance your proficiency.
6. Distributive Property with Variables
The distributive property is equally applicable when dealing with algebraic expressions involving variables.
6.1 Applying the Distributive Property to Algebraic Expressions
When variables are involved, the distributive property helps simplify expressions by multiplying the term outside the parentheses with each term inside.
Example: 4 (x + 3)
-
Distribute:
- 4 × x + 4 × 3
6.2 Simplifying Expressions
Simplify the expression by performing the multiplication.
-
Multiply:
- 4 × x = 4x
- 4 × 3 = 12
-
Combine:
- 4x + 12
Therefore, 4 (x + 3) simplifies to 4x + 12.
6.3 Example with Subtraction
Consider the expression: 2 (y – 5)
-
Distribute:
- 2 × y – 2 × 5
-
Multiply:
- 2 × y = 2y
- 2 × 5 = 10
-
Combine:
- 2y – 10
Therefore, 2 (y – 5) simplifies to 2y – 10.
7. Distributive Property of Division
The distributive property can also be applied to division, but it requires careful handling.
7.1 Breaking Down the Dividend
When dividing, the dividend (the number being divided) can be broken down into parts that are easily divisible by the divisor.
Example: 144 ÷ 6
-
Break Down:
- 144 can be broken down into 60 + 60 + 24
-
Divide:
- (60 ÷ 6) + (60 ÷ 6) + (24 ÷ 6)
- = 10 + 10 + 4
- = 24
Therefore, 144 ÷ 6 = 24
7.2 Important Considerations
- Divisor Integrity: Do not break down the divisor. Only break down the dividend.
- Divisibility: Ensure that each part of the dividend is divisible by the divisor.
For example, breaking 144 ÷ 6 into (50 + 50 + 44) ÷ 6 would not work because 50 and 44 are not divisible by 6.
7.3 When Not to Use
The distributive property is not effective if the parts of the dividend are not easily divisible by the divisor.
8. Common Mistakes to Avoid
To master the distributive property, be aware of common pitfalls.
8.1 Forgetting to Distribute to All Terms
Ensure that the multiplier is distributed to every term inside the parentheses.
Incorrect: 3 (x + 2) = 3x + 2 (missing the distribution to 2)
Correct: 3 (x + 2) = 3x + 6
8.2 Misunderstanding Signs
Pay close attention to signs, especially with subtraction and negative numbers.
Incorrect: -2 (y – 3) = -2y – 6 (incorrect sign for the last term)
Correct: -2 (y – 3) = -2y + 6
8.3 Incorrect Order of Operations
Follow the correct order of operations (PEMDAS/BODMAS) when simplifying expressions.
Incorrect: 4 + 2 (x + 1) = 6 (x + 1) (adding 4 and 2 before distributing)
Correct: 4 + 2 (x + 1) = 4 + 2x + 2 = 2x + 6
8.4 Tips for Avoiding Mistakes
- Write It Out: Explicitly write out each distribution step to minimize errors.
- Double-Check Signs: Always double-check the signs, especially when dealing with negative numbers.
- Practice Regularly: Consistent practice helps solidify understanding and reduces the chance of errors.
9. Advanced Applications
The distributive property is a cornerstone for more complex mathematical concepts.
9.1 Expanding Polynomial Expressions
The distributive property is essential for expanding polynomial expressions.
Example: (x + 2) (x + 3)
-
Distribute:
- x (x + 3) + 2 (x + 3)
-
Expand:
- x^2 + 3x + 2x + 6
-
Combine Like Terms:
- x^2 + 5x + 6
9.2 Simplifying Algebraic Equations
It is used to simplify algebraic equations, making them easier to solve.
Example: 3 (2x + 1) – 2 (x – 4) = 15
-
Distribute:
- 6x + 3 – 2x + 8 = 15
-
Combine Like Terms:
- 4x + 11 = 15
-
Solve for x:
- 4x = 4
- x = 1
9.3 Factoring
Factoring is the reverse of distribution. It involves identifying a common factor and extracting it.
Example: 6x + 9
-
Identify Common Factor:
- The common factor is 3
-
Factor Out:
- 3 (2x + 3)
10. Practice Problems
Reinforce your understanding with these practice problems.
10.1 Solve the Following Expressions
- 5 × (3 + 4)
- 8 × (7 – 2)
- 3 (x + 5)
- -2 (y – 4)
- (a + 2) (a + 1)
10.2 Solutions
- 5 × (3 + 4) = 5 × 7 = 35 OR (5 × 3) + (5 × 4) = 15 + 20 = 35
- 8 × (7 – 2) = 8 × 5 = 40 OR (8 × 7) – (8 × 2) = 56 – 16 = 40
- 3 (x + 5) = 3x + 15
- -2 (y – 4) = -2y + 8
- (a + 2) (a + 1) = a^2 + a + 2a + 2 = a^2 + 3a + 2
10.3 Additional Resources
- Online Quizzes: Test your knowledge with interactive quizzes.
- Worksheets: Practice with downloadable worksheets.
- Tutoring: Seek personalized help from a math tutor.
11. FAQs About the Distributive Property
11.1 Common Questions Answered
Q1: Does the distributive property apply to division?
Yes, but only by breaking down the dividend, ensuring each part is divisible by the divisor.
Q2: What is the rule for the distributive property?
A (B + C) = AB + AC for addition, and A (B – C) = AB – AC for subtraction.
Q3: How can the distributive property help in solving complex questions?
It simplifies complex expressions by distributing terms, making the problem more manageable.
Q4: Can parentheses be removed after distributing?
Yes, distributing removes the parentheses, allowing you to simplify the expression further.
Q5: How do we use the distributive property with equations?
Use it to expand terms or simplify expressions in the equation, making it easier to solve.
11.2 Still Have Questions?
If you have more questions, visit what.edu.vn to ask anything and get free answers from our community of experts. Our platform is designed to provide you with quick, reliable solutions to all your queries.
12. History and Evolution of the Distributive Property
12.1 Ancient Roots
The concept of distribution in mathematics dates back to ancient civilizations, where early mathematicians recognized the importance of breaking down complex calculations into simpler parts. While not explicitly formulated as the distributive property we know today, the underlying ideas were present in various forms.
Early Examples
- Egyptian Multiplication: Egyptians used a method of multiplication based on repeated doubling and addition. This can be seen as an early form of distributing multiplication over addition.
- Babylonian Algebra: Babylonians developed algebraic techniques that implicitly used distribution when solving equations.
12.2 Formalization in Classical Mathematics
The formalization of the distributive property as a distinct mathematical concept emerged during the development of algebra in classical mathematics.
Greek Contributions
- Euclid: In his book “Elements,” Euclid presented geometric proofs that indirectly illustrated the distributive property. While he didn’t state it algebraically, his geometric interpretations showed an understanding of how areas and volumes could be distributed.
Indian Mathematics
- Brahmagupta: In the 7th century CE, Indian mathematician Brahmagupta provided rules for working with positive and negative numbers, which implicitly used distribution in algebraic manipulations.
12.3 Development in Islamic Mathematics
Islamic mathematicians played a crucial role in the formal development of algebra and, consequently, the distributive property.
Al-Khwarizmi
- Algebraic Techniques: Al-Khwarizmi, often called the “father of algebra,” introduced systematic algebraic methods in the 9th century. His work involved manipulating equations in ways that depended on distribution.
Later Islamic Scholars
- Refinement: Later scholars refined these techniques, providing more explicit formulations of algebraic principles, including distribution.
12.4 European Renaissance and Beyond
The European Renaissance saw a renewed interest in classical mathematics, leading to further development and formalization of algebraic concepts.
Early Modern Period
- Symbolic Algebra: The introduction of symbolic algebra by mathematicians like François Viète allowed for more abstract and general statements of the distributive property.
- Formal Definition: By the 17th century, the distributive property was recognized and stated in a form similar to what we use today.
Modern Mathematics
- Abstract Algebra: In modern abstract algebra, the distributive property is a fundamental axiom defining rings and fields, which are essential structures in advanced mathematics.
12.5 Timeline of Key Developments
| Period | Civilization/Mathematician | Contribution |
|---|---|---|
| Ancient Egypt | Egyptians | Used repeated doubling and addition, an early form of distributing multiplication over addition. |
| Ancient Babylonia | Babylonians | Developed algebraic techniques that implicitly used distribution. |
| Ancient Greece | Euclid | Presented geometric proofs illustrating distribution indirectly in “Elements.” |
| 7th Century CE | Brahmagupta | Provided rules for positive and negative numbers, implicitly using distribution. |
| 9th Century CE | Al-Khwarizmi | Introduced systematic algebraic methods that depended on distribution. |
| European Renaissance | Various Mathematicians | Formalized symbolic algebra, leading to explicit statements of the distributive property. |
| 17th Century | European Mathematicians | Recognized and stated the distributive property in a form similar to modern usage. |
| Modern Abstract Algebra | Modern Mathematicians | The distributive property became a fundamental axiom defining rings and fields in abstract algebra. |

12.6 Impact and Significance
The distributive property has had a profound impact on the development of mathematics:
- Foundation of Algebra: It is a foundational principle in algebra, allowing for the simplification and manipulation of algebraic expressions and equations.
- Advanced Mathematics: It extends to more advanced areas like calculus, abstract algebra, and mathematical analysis.
- Practical Applications: Its applications are widespread, from basic arithmetic to complex engineering calculations.
13. The Distributive Property in Everyday Life
13.1 Practical Scenarios
The distributive property isn’t just a theoretical concept; it has numerous practical applications in everyday life. Here are some common scenarios where you might use it without even realizing it:
Grocery Shopping
Imagine you’re buying multiple items of the same type, and each item has a base price plus tax.
- Scenario: You want to buy 5 cans of soup. Each can costs $2, and the sales tax is $0.10 per can.
- Calculation:
- Total cost per can: $2 + $0.10 = $2.10
- Using distributive property: 5 × ($2 + $0.10) = (5 × $2) + (5 × $0.10) = $10 + $0.50 = $10.50
- Explanation: You multiply 5 by both the price and the tax, then add the results to find the total cost.
Calculating Expenses
When budgeting or calculating expenses, you might use the distributive property to simplify your calculations.
- Scenario: You spend $15 per day on weekdays (Monday to Friday) and $25 per day on weekends (Saturday and Sunday).
- Calculation:
- Total weekdays: 5
- Total weekends: 2
- Using distributive property: 5 × $15 + 2 × $25 = $75 + $50 = $125
- Explanation: You calculate the total spending for weekdays and weekends separately, then add them up to find the total weekly spending.
Home Improvement Projects
Calculating materials for home improvement projects often involves using the distributive property.
- Scenario: You want to paint three rooms in your house. Each room requires 2 gallons of paint, and you also need 1 gallon for touch-ups.
- Calculation:
- Total paint needed per room: 2 + 1 = 3 gallons
- Using distributive property: 3 × (2 + 1) = (3 × 2) + (3 × 1) = 6 + 3 = 9 gallons
- Explanation: You multiply the number of rooms by the sum of the paint needed for each room to find the total amount of paint required.
Cooking and Baking
When adjusting recipes, the distributive property can help scale ingredients correctly.
- Scenario: A recipe calls for 2 cups of flour and 1 cup of sugar. You want to triple the recipe.
- Calculation:
- Using distributive property: 3 × (2 + 1) = (3 × 2) + (3 × 1) = 6 cups of flour + 3 cups of sugar
- Explanation: You multiply the amount of each ingredient by the scaling factor to adjust the recipe.
Financial Planning
Calculating investment returns or loan payments can also involve the distributive property.
- Scenario: You invest $1,000 in an account that earns 5% interest annually. You also contribute an additional $200 each year.
- Calculation:
- Total investment: $1,000 + $200 = $1,200
- Using distributive property for interest calculation: ($1,000 + $200) × 0.05 = ($1,000 × 0.05) + ($200 × 0.05) = $50 + $10 = $60
- Explanation: You calculate the interest earned on both the initial investment and the additional contribution, then add them up to find the total interest earned.
Event Planning
When organizing events, you can use the distributive property to calculate costs and quantities efficiently.
- Scenario: You’re planning a party for 30 guests. Each guest will need 2 slices of pizza and 3 drinks.
- Calculation:
- Total slices of pizza: 30 × 2 = 60 slices
- Total drinks: 30 × 3 = 90 drinks
- Using distributive property to find total needs: 30 × (2 + 3) = (30 × 2) + (30 × 3) = 60 + 90 = 150 items
- Explanation: You multiply the number of guests by the sum of the pizza slices and drinks needed per guest to find the total requirements.
13.2 Table of Scenarios
| Scenario | Example | Distributive Property Application | Calculation |
|---|---|---|---|
| Grocery Shopping | Buying 5 cans of soup at $2 each, plus $0.10 tax per can. | 5 × ($2 + $0.10) = (5 × $2) + (5 × $0.10) | $10 + $0.50 = $10.50 |
| Calculating Expenses | Spending $15/day on weekdays and $25/day on weekends. | (5 × $15) + (2 × $25) | $75 + $50 = $125 |
| Home Improvement | Painting 3 rooms, each needing 2 gallons of paint and 1 gallon for touch-ups. | 3 × (2 + 1) = (3 × 2) + (3 × 1) | 6 + 3 = 9 gallons |
| Cooking and Baking | Tripling a recipe that calls for 2 cups of flour and 1 cup of sugar. | 3 × (2 + 1) = (3 × 2) + (3 × 1) | 6 cups of flour + 3 cups of sugar |
| Financial Planning | Investing $1,000 with 5% interest and contributing an additional $200 annually. | ($1,000 + $200) × 0.05 = ($1,000 × 0.05) + ($200 × 0.05) | $50 + $10 = $60 |
| Event Planning | Planning a party for 30 guests, each needing 2 slices of pizza and 3 drinks. | 30 × (2 + 3) = (30 × 2) + (30 × 3) | 60 + 90 = 150 items |
13.3 Why It Matters
Understanding and applying the distributive property makes everyday calculations easier and more efficient. Whether you’re managing your finances, planning events, or working on home improvement projects, the distributive property is a valuable tool.
14. The Distributive Property and Mental Math
14.1 Strategies for Mental Calculation
The distributive property is a powerful tool for performing mental math calculations quickly and accurately. By breaking down numbers into more manageable parts, you can simplify complex problems and solve them in your head.
Breaking Down Numbers
One of the key strategies for using the distributive property in mental math is breaking down numbers into easier components.
- Example: Calculate 6 × 13 mentally.
- Break down 13 into 10 + 3.
- Apply distributive property: 6 × (10 + 3) = (6 × 10) + (6 × 3)
- Calculate: 60 + 18 = 78
- Therefore, 6 × 13 = 78
Using Compatible Numbers
Choose numbers that are easy to work with mentally to simplify calculations.
- Example: Calculate 8 × 17 mentally.
- Think of 17 as 20 – 3.
- Apply distributive property: 8 × (20 – 3) = (8 × 20) – (8 × 3)
- Calculate: 160 – 24 = 136
- Therefore, 8 × 17 = 136
Adjusting and Compensating
Adjust numbers to make them easier to multiply, and then compensate for the adjustment.
- Example: Calculate 9 × 26 mentally.
- Think of 9 as 10 – 1.
- Apply distributive property: (10 – 1) × 26 = (10 × 26) – (1 × 26)
- Calculate: 260 – 26 = 234
- Therefore, 9 × 26 = 234
14.2 Examples and Techniques
Here are more examples of how to use the distributive property for mental math:
Multiplying by Numbers Close to 10
When multiplying by numbers like 9, 11, or 12, use the distributive property to simplify.
- Example: Calculate 11 × 34 mentally.
- Think of 11 as 10 + 1.
- Apply distributive property: (10 + 1) × 34 = (10 × 34) + (1 × 34)
- Calculate: 340 + 34 = 374
- Therefore, 11 × 34 = 374
Multiplying by Numbers Close to 100
Similarly, when multiplying by numbers close to 100, use the distributive property to simplify.
- Example: Calculate 99 × 15 mentally.
- Think of 99 as 100 – 1.
- Apply distributive property: (100 – 1) × 15 = (100 × 15) – (1 × 15)
- Calculate: 1500 – 15 = 1485
- Therefore, 99 × 15 = 1485
Combining Techniques
Combine multiple techniques to make calculations even easier.
- Example: Calculate 12 × 23 mentally.
- Think of 12 as 10 + 2.
- Break down 23 into 20 + 3.
- Apply distributive property: (10 + 2) × (20 + 3) = (10 × 20) + (10 × 3) + (2 × 20) + (2 × 3)
- Calculate: 200 + 30 + 40 + 6 = 276
- Therefore, 12 × 23 = 276
14.3 Practice Exercises
Try these practice exercises to improve your mental math skills using the distributive property:
- Calculate 7 × 14 mentally.
- Calculate 9 × 18 mentally.
- Calculate 11 × 25 mentally.
- Calculate 99 × 12 mentally.
- Calculate 13 × 21 mentally.
14.4 Benefits of Using Distributive Property for Mental Math
Using the distributive property for mental math offers several benefits:
- Simplifies Calculations: Breaks down complex problems into simpler steps.
- Improves Accuracy: Reduces the chance of errors by managing smaller numbers.
- Enhances Speed: Allows for quicker calculations once the technique is mastered.
- Increases Confidence: Boosts confidence in your mental math abilities.
14.5 Tips for Success
- Practice Regularly: Consistent practice is key to mastering mental math.
- Start Simple: Begin with easier problems and gradually increase difficulty.
- Visualize: Try to visualize the numbers and steps in your mind.
- Use Real-Life Scenarios: Apply mental math techniques to everyday situations.
14.6 Summary Table: Techniques for Mental Math
| Technique | Description | Example |
|---|---|---|
| Breaking Down Numbers | Decompose numbers into easier components. | 6 × 13 = 6 × (10 + 3) = 60 + 18 = 78 |
| Using Compatible Numbers | Choose numbers that are easy to work with mentally. | 8 × 17 = 8 × (20 – 3) = 160 – 24 = 136 |
| Adjusting and Compensating | Adjust numbers to make them easier to multiply, then compensate. | 9 × 26 = (10 – 1) × 26 = 260 – 26 = 234 |
| Multiplying by Numbers Close to 10 | Use the distributive property to simplify multiplications by numbers like 9, 11, or 12. | 11 × 34 = (10 + 1) × 34 = 340 + 34 = 374 |
| Multiplying by Numbers Close to 100 | Use the distributive property to simplify multiplications by numbers close to 100. | 99 × 15 = (100 – 1) × 15 = 1500 – 15 = 1485 |
By mastering these techniques, you can significantly improve your mental math skills and tackle calculations with ease.
15. Resources for Further Learning
15.1 Books and Publications
Recommended Books
- “Pre-Algebra for Dummies” by Mary Jane Sterling: This book provides a comprehensive overview of pre-algebra concepts, including the distributive property, with clear explanations and numerous practice problems.
- “Algebra I for Dummies” by Mary Jane Sterling: A step-by-step guide to algebra basics, offering detailed explanations and examples of how to use the distributive property in algebraic expressions.
- “The ম্যাথ Book: From Pythagoras to the 57th Dimension, 250 Milestones in the History of Mathematics” by Clifford A. Pickover: This book explores various mathematical concepts throughout history, providing context and insights into the development of fundamental principles like the distributive property.
Academic Journals
- “The Mathematics Teacher”: Published by the National Council of Teachers of Mathematics (NCTM), this journal features articles on teaching strategies, mathematical concepts, and classroom activities related to algebra and pre-algebra topics.
- “The American Mathematical Monthly”: This journal includes articles on a wide range of mathematical topics, including discussions on algebraic structures and properties relevant to the distributive property.
15.2 Online Courses and Tutorials
Khan Academy
- Algebra Basics: Khan Academy offers a comprehensive course on algebra basics, covering the distributive property with video lessons, practice exercises, and quizzes.
- Pre-Algebra: This course provides a foundational understanding of pre-algebra concepts, including detailed explanations and examples of the distributive property.
Coursera
- “Introduction to Algebra” by University of California, Irvine: This course covers the fundamental concepts of algebra, including the distributive property, with video lectures and interactive assignments.
edX
- “Algebra I” by Davidson College: This course offers a thorough introduction to algebra, with