The square root of 64 is a common mathematical inquiry, and WHAT.EDU.VN is here to provide a clear explanation. Understanding the square root of 64 is essential for various mathematical applications. Explore square root, perfect square and rational number with us.
1. Understanding the Square Root of 64
The square root of a number is a value that, when multiplied by itself, equals the original number. In simpler terms, if you have a square, the square root is the length of one of its sides, given the area. So, what number, when multiplied by itself, gives you 64?
The square root of 64 is 8.
This is because 8 multiplied by 8 equals 64 (8 x 8 = 64). We can represent this mathematically as:
√64 = 8
Therefore, 64 is a perfect square.
2. Why is Understanding Square Roots Important?
Square roots are not just abstract mathematical concepts. They have practical applications in various fields, including:
- Geometry: Calculating the sides of squares and other shapes.
- Physics: Determining distances, velocities, and accelerations.
- Engineering: Designing structures and calculating stress and strain.
- Computer Science: Developing algorithms and solving computational problems.
Understanding square roots can make these calculations more intuitive and efficient.
3. Methods for Finding the Square Root of 64
While knowing that the square root of 64 is 8 is helpful, understanding how to find it is even more important. Here are a couple of common methods:
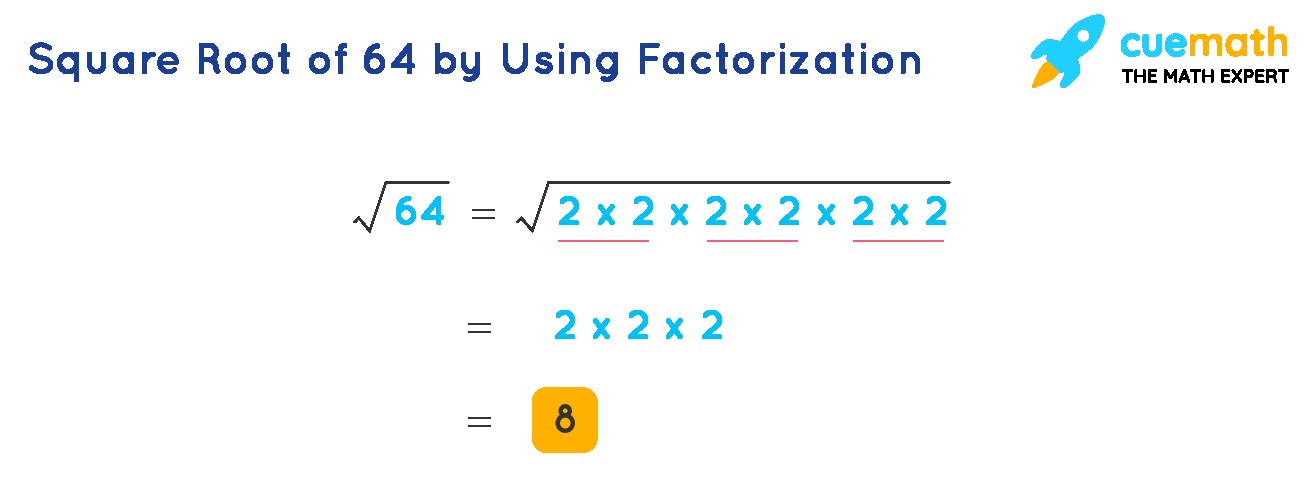
3.1 Prime Factorization Method
This method involves breaking down the number into its prime factors. Prime factors are numbers that are only divisible by 1 and themselves (e.g., 2, 3, 5, 7, 11).
- Find the prime factorization of 64: 64 = 2 x 2 x 2 x 2 x 2 x 2
- Group the factors into pairs: (2 x 2) x (2 x 2) x (2 x 2)
- Take one number from each pair: 2 x 2 x 2
- Multiply the numbers together: 2 x 2 x 2 = 8
Therefore, the square root of 64 is 8.
3.2 Repeated Subtraction Method
This method involves repeatedly subtracting consecutive odd numbers from the original number until you reach zero. The number of subtractions it takes to reach zero is the square root.
- Start with 64.
- Subtract 1: 64 – 1 = 63
- Subtract 3: 63 – 3 = 60
- Subtract 5: 60 – 5 = 55
- Subtract 7: 55 – 7 = 48
- Subtract 9: 48 – 9 = 39
- Subtract 11: 39 – 11 = 28
- Subtract 13: 28 – 13 = 15
- Subtract 15: 15 – 15 = 0
It took 8 subtractions to reach zero, therefore the square root of 64 is 8.
While this method works, it’s not very efficient for larger numbers.
4. Is the Square Root of 64 Rational or Irrational?
Understanding the difference between rational and irrational numbers is crucial.
- Rational numbers can be expressed as a fraction p/q, where p and q are integers and q is not zero. They can be terminating decimals (e.g., 0.5) or repeating decimals (e.g., 0.333…).
- Irrational numbers cannot be expressed as a fraction and have non-repeating, non-terminating decimal representations (e.g., π, √2).
Since the square root of 64 is 8, which can be expressed as the fraction 8/1, it is a rational number.
5. What About Negative Square Roots?
While 8 x 8 = 64, it’s also true that (-8) x (-8) = 64. This means that 64 has two square roots: a positive square root (8) and a negative square root (-8).
When we use the radical symbol (√), we generally refer to the principal square root, which is the positive square root. However, when solving equations, it’s important to consider both the positive and negative square roots.
6. Square Root of 64 in Real-World Applications
Let’s look at some practical examples of how the square root of 64 might be used:
- Gardening: You’re building a square garden bed with an area of 64 square feet. To determine how long each side should be, you need to find the square root of 64, which is 8 feet.
- Construction: An architect is designing a square courtyard with an area of 64 square meters. To ensure the courtyard fits the design, they need to know that each side will be 8 meters long.
- Art: An artist is creating a square mosaic with 64 individual tiles. To arrange the tiles in a perfect square, they need to know that they should arrange them in an 8×8 grid.
7. Beyond the Basics: Exploring Related Concepts
Understanding the square root of 64 is a gateway to exploring other related mathematical concepts:
- Squares: The square of a number is the result of multiplying it by itself (e.g., the square of 8 is 64).
- Cubes: The cube of a number is the result of multiplying it by itself three times (e.g., the cube of 4 is 64).
- Perfect Cubes: A perfect cube is a number that can be obtained by cubing an integer (e.g., 64 is a perfect cube because 4 x 4 x 4 = 64).
- Higher Roots: Just as you can find the square root of a number, you can also find the cube root, fourth root, and so on.
8. Common Mistakes to Avoid
When working with square roots, here are some common mistakes to watch out for:
- Forgetting the negative square root: Remember that every positive number has two square roots, a positive and a negative one.
- Confusing square root with division: The square root is not the same as dividing a number by 2.
- Incorrectly applying the order of operations: Make sure to calculate square roots before performing addition or subtraction.
9. The Importance of Practice
Like any mathematical skill, mastering square roots requires practice. Work through examples, solve problems, and don’t be afraid to make mistakes. The more you practice, the more confident you’ll become.
10. Square Root of 64: Solved Examples
Let’s reinforce our understanding with some solved examples:
Example 1:
A square tile has an area of 64 square inches. What is the length of one side of the tile?
Solution:
The length of one side is the square root of the area. Therefore, the side length is √64 = 8 inches.
Example 2:
A square garden has a perimeter of 32 feet. What is the area of the garden?
Solution:
First, find the length of one side by dividing the perimeter by 4: 32 feet / 4 = 8 feet. Then, find the area by squaring the side length: 8 feet x 8 feet = 64 square feet.
Example 3:
Solve for x: x² = 64
Solution:
Take the square root of both sides: √x² = √64. Therefore, x = 8 or x = -8.
11. Square Root of 64: Interactive Questions
Test your knowledge with these interactive questions:
Question 1:
A square poster has an area of 64 square centimeters. What is the length of its sides?
Answer: 8 centimeters
Question 2:
If the side of a square is 8 meters, what is its area?
Answer: 64 square meters
Question 3:
What is the value of √64 + 5?
Answer: 13
12. Exploring Square Roots Beyond 64
The principles we’ve discussed for finding the square root of 64 can be applied to finding the square roots of other numbers as well. While some numbers have perfect square roots (like 9, 16, 25), others have irrational square roots that can only be approximated.
Understanding the concepts of perfect squares, prime factorization, and estimation techniques will help you tackle a wider range of square root problems.
13. Advanced Concepts: Complex Numbers and Square Roots
While we’ve focused on real numbers, the concept of square roots extends to complex numbers as well. Complex numbers involve the imaginary unit “i,” where i² = -1.
Every complex number has two square roots, which are also complex numbers. Finding the square roots of complex numbers involves using complex number arithmetic and DeMoivre’s Theorem.
14. The History of Square Roots
The concept of square roots has been around for centuries. Ancient civilizations, such as the Babylonians and Egyptians, used square roots in their calculations for land surveying, construction, and astronomy.
The symbol “√” was first used in the 16th century. Over time, mathematicians developed more sophisticated methods for calculating square roots, including algorithms and numerical approximations.
15. Square Root of 64: A Foundation for Further Learning
Understanding the square root of 64 is not just about memorizing a number. It’s about developing a deeper understanding of mathematical concepts, problem-solving skills, and the connections between different areas of mathematics.
By mastering this fundamental concept, you’ll be well-prepared to tackle more advanced topics in algebra, geometry, calculus, and beyond.
16. Memorization Techniques for Square Roots
Memorizing the square roots of perfect squares can be helpful for quick calculations and mental math. Here are some techniques to help you memorize:
- Flashcards: Create flashcards with the perfect square on one side and its square root on the other.
- Patterns: Look for patterns in the sequence of square roots (1, 4, 9, 16, 25, 36, 49, 64, 81, 100).
- Mnemonics: Create memorable phrases or rhymes to associate the perfect square with its square root.
- Practice: Regularly test yourself on the square roots you’re trying to memorize.
17. Estimation Techniques for Square Roots
When dealing with numbers that don’t have perfect square roots, estimation techniques can be valuable. Here’s how to estimate the square root of a number:
- Identify the nearest perfect squares: Find the two perfect squares that the number falls between.
- Determine the position: Determine where the number falls between the two perfect squares.
- Estimate the square root: Estimate the square root based on its position between the square roots of the nearest perfect squares.
For example, to estimate the square root of 70:
- The nearest perfect squares are 64 (√64 = 8) and 81 (√81 = 9).
- 70 is closer to 64 than to 81.
- Therefore, the square root of 70 is approximately 8.3.
18. Calculators and Technology
While it’s important to understand the concepts and methods behind square roots, calculators and computer software can be valuable tools for performing calculations quickly and accurately.
Most calculators have a square root function (usually denoted by the symbol “√”). Simply enter the number and press the square root button to find its square root.
Spreadsheet software like Microsoft Excel and Google Sheets also have square root functions that can be used for calculations and data analysis.
19. Engaging Activities for Learning Square Roots
Learning square roots doesn’t have to be dry and boring. Here are some engaging activities to make the learning process more fun:
- Square Root Bingo: Create bingo cards with square roots on them and call out the corresponding perfect squares.
- Square Root Scavenger Hunt: Hide objects around the room with square roots attached to them and have students find the objects and identify the square roots.
- Square Root Puzzles: Create puzzles where students have to match perfect squares with their corresponding square roots.
- Real-World Projects: Have students design and build structures that require them to use square roots in their calculations.
20. Frequently Asked Questions (FAQs) About Square Root of 64
Let’s address some frequently asked questions about the square root of 64:
Q: What is the symbol for square root?
A: The symbol for square root is “√”.
Q: Is there a difference between the square root and the principal square root?
A: Yes, the square root of a number can be positive or negative, while the principal square root is the positive square root.
Q: How do I find the square root of a fraction?
A: To find the square root of a fraction, take the square root of the numerator and the square root of the denominator separately.
Q: Can I find the square root of a negative number?
A: No, the square root of a negative number is not a real number. It is an imaginary number.
Q: What is the square root of zero?
A: The square root of zero is zero.
21. The Role of WHAT.EDU.VN in Your Learning Journey
At WHAT.EDU.VN, we understand that finding quick, free answers to your questions can be challenging. You might not know who to ask or where to look for reliable information. You might worry about the cost of consultations or struggle to find a user-friendly platform for your inquiries.
That’s why we’ve created WHAT.EDU.VN: a free platform where you can ask any question and receive fast, accurate answers. Our goal is to provide you with easy-to-understand and helpful information, connecting you with a community of knowledgeable individuals who are ready to share their expertise.
22. Why Choose WHAT.EDU.VN?
- Free Access: Ask any question without worrying about fees or subscriptions.
- Quick Responses: Get answers promptly so you can keep moving forward.
- Accurate Information: Rely on knowledgeable contributors for reliable information.
- User-Friendly Platform: Easily navigate our site and find the answers you need.
- Community Support: Connect with others who are curious and eager to learn.
23. Real-World Scenarios and the Need for Quick Answers
Imagine you’re a student working on a math assignment and you’re stuck on a problem involving square roots. You need a quick answer to understand the concept and complete your work on time.
Or, perhaps you’re a professional working on a design project that requires precise measurements. You need to calculate the square root of a number to ensure the accuracy of your design.
In these situations, WHAT.EDU.VN can be your go-to resource for finding the answers you need, when you need them.
24. Testimonials and Success Stories
“I was struggling with a math problem and needed help fast. WHAT.EDU.VN provided me with a clear and concise explanation that helped me understand the concept and finish my assignment on time.” – Sarah M.
“As a designer, I often need to perform complex calculations. WHAT.EDU.VN is a valuable resource that provides me with quick and accurate answers, saving me time and ensuring the quality of my work.” – John B.
25. Embracing Curiosity and Lifelong Learning
At WHAT.EDU.VN, we believe that curiosity is the key to lifelong learning. We encourage you to ask questions, explore new topics, and never stop seeking knowledge.
Whether you’re a student, a professional, or simply someone who is curious about the world around you, WHAT.EDU.VN is here to support your learning journey.
26. Conclusion: The Square Root of 64 and Beyond
Understanding the square root of 64 is a fundamental mathematical concept that has practical applications in various fields. By mastering this concept and exploring related topics, you’ll be well-prepared to tackle more advanced mathematical challenges.
Remember, WHAT.EDU.VN is here to support your learning journey by providing you with quick, free answers to your questions. Don’t hesitate to reach out and ask us anything!
27. Call to Action: Ask Your Questions Today
Do you have any questions about square roots, mathematics, or any other topic? Visit WHAT.EDU.VN today and ask your questions for free!
Our team of experts and knowledgeable community members are ready to provide you with the answers you need.
Address: 888 Question City Plaza, Seattle, WA 98101, United States
WhatsApp: +1 (206) 555-7890
Website: WHAT.EDU.VN
28. Final Thoughts: Empowering Knowledge Seekers
We believe that everyone deserves access to knowledge and answers. That’s why we’re committed to providing a free, user-friendly platform where you can ask any question and receive fast, accurate responses.
Join our community of curious learners and let us help you on your journey to knowledge and understanding. We provide services to cung cấp một nền tảng miễn phí để đặt bất kỳ câu hỏi nào, trả lời câu hỏi một cách nhanh chóng và chính xác, cung cấp thông tin dễ hiểu và hữu ích, kết nối người dùng với cộng đồng để trao đổi kiến thức và cung cấp dịch vụ tư vấn miễn phí cho các vấn đề đơn giản.
Don’t hesitate – ask your questions on what.edu.vn today! Let us help you unlock the answers you seek and empower you with the knowledge you need.