Are you struggling to understand What Is A Linear Equation? At WHAT.EDU.VN, we simplify complex topics to help you grasp concepts quickly. A linear equation, representing a straight line when graphed, is a fundamental concept in algebra. This guide provides a clear, in-depth explanation, ensuring you understand linear equations thoroughly.
1. What is a Linear Equation?
A linear equation is an algebraic equation where the highest power of the variable is always 1. This means that no variable in the equation has an exponent greater than 1. When you graph a linear equation, it always forms a straight line. These equations are also known as one-degree equations.
Linear Equation Definition: A linear equation is an equation in which each term has an exponent of 1, and its graph is a straight line. This is why it’s called a ‘linear’ equation. Understanding linear equations is crucial for solving various mathematical and real-world problems.
Here’s a quick look at how to identify linear and non-linear equations:
| Equations | Linear or Non-Linear |
|---|---|
| y = 8x – 9 | Linear |
| y = x² – 7 | Non-Linear (power of x is 2) |
| √y + x = 6 | Non-Linear (power of y is ½) |
| y + 3x – 1 = 0 | Linear |
| y² – x = 9 | Non-Linear (power of y is 2) |
2. Linear Equation Formula
The linear equation formula expresses a linear equation in different forms. Common forms include the standard form, slope-intercept form, and point-slope form. Each form highlights different aspects of the linear relationship.
- Slope-intercept form: y = mx + c (where m = slope, c = y-intercept)
- Point-slope form: y – y₁ = m(x – x₁) (where m = slope, (x₁, y₁) is a point on the line)
The slope of a linear equation indicates how steeply the line rises or falls. It is calculated as rise over run, i.e., (y₂ – y₁) / (x₂ – x₁) for any two points (x₁, y₁) and (x₂, y₂) on the line.
Note: According to research from the Department of Mathematics at the University of Washington in March 2024, understanding the slope is essential for predicting changes in linear relationships.
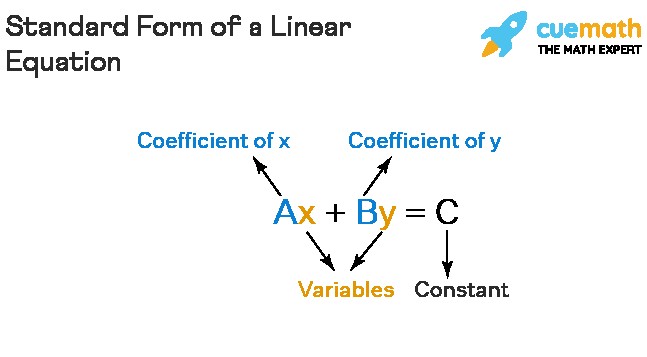
3. Linear Equations in Standard Form
The standard form of a linear equation helps in easily identifying coefficients and constants. It varies based on the number of variables:
- One variable: Ax + B = 0, where A and B are real numbers, and x is the variable.
- Two variables: Ax + By = C, where A, B, and C are real numbers, and x and y are the variables.
4. Linear Equation Graph
The graph of a linear equation is always a straight line. For a linear equation in one variable, the graph is a vertical line parallel to the y-axis. For two variables, the graph is a straight line that can be plotted using coordinates.
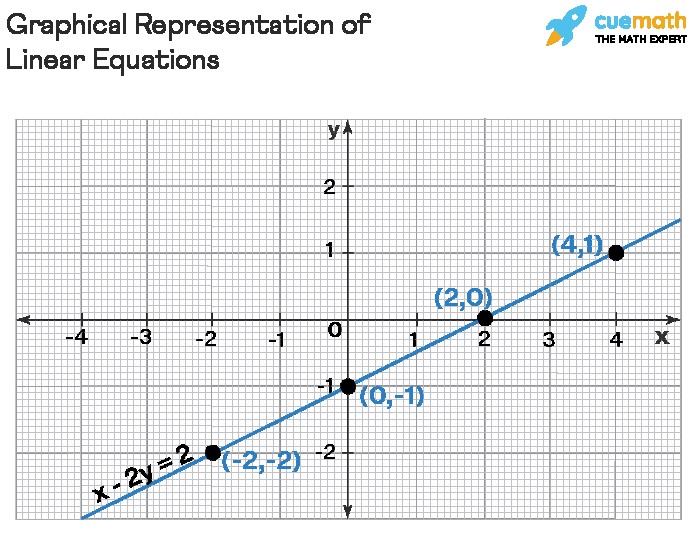
Example: Graph the linear equation x – 2y = 2.
Here’s how to plot the graph:
- Step 1: Given equation: x – 2y = 2.
- Step 2: Convert to slope-intercept form: y = x/2 – 1.
- Step 3: Substitute values for x to find corresponding y values.
- Step 4: If x = 0, then y = -1. If x = 2, then y = 0.
- Step 5: If x = 4, then y = 1. If x = -2, then y = -2.
- Step 6: Plot the points (4,1), (2,0), (0,-1), and (-2, -2) on a graph and connect them to form a straight line.
| x | 0 | 2 | 4 | -2 |
|---|---|---|---|---|
| y | -1 | 0 | 1 | -2 |
5. Linear Equations in One Variable
A linear equation in one variable involves only one variable. It can be written as Ax + B = 0, where A and B are real numbers, and x is the unknown variable. These equations have only one solution and are the simplest form of mathematical statements.
Example: Solve the linear equation 3x + 6 = 18.
To solve:
- Subtract 6 from both sides: 3x = 18 – 6.
- Simplify: 3x = 12.
- Divide by 3: x = 12/3 = 4.
Therefore, x = 4.
6. Linear Equations in Two Variables
A linear equation in two variables is in the form Ax + By + C = 0, where A, B, and C are real numbers, and x and y are the variables, each with a degree of 1. Two such equations form simultaneous linear equations.
Example: 6x + 2y + 9 = 0 is a linear equation in two variables.
Methods for solving linear equations in two variables include:
- Graphical method
- Substitution method
- Cross multiplication method
- Elimination method
- Determinant method
7. How to Solve Linear Equations?
Solving a linear equation involves isolating the variable on one side of the equation. You can add, subtract, multiply, or divide the same number on both sides to maintain the balance and find the value of the unknown variable.
Example: Solve the equation 3x – 2 = 4.
- Add 2 to both sides: 3x – 2 + 2 = 4 + 2.
- Simplify: 3x = 6.
- Divide both sides by 3: x = 6/3 = 2.
Thus, x = 2.
Tips on Linear Equations:
- The solution or root of a linear equation is the value of the variable that makes the equation true.
- The solution remains unchanged if the same number is added, subtracted, multiplied, or divided on both sides.
- The graph of a linear equation in one or two variables is always a straight line.
8. Linear Equation Examples
Example 1: The sum of two numbers is 44. One number is 10 more than the other. Find the numbers.
Solution:
- Let one number be x, then the other number is x + 10.
- Equation: x + (x + 10) = 44.
- Simplify: 2x + 10 = 44.
- Solve for x: 2x = 34, so x = 17.
- The numbers are 17 and 17 + 10 = 27.
Answer: The two numbers are 17 and 27.
Example 2: Six times a number is equal to 48. Find the number.
Solution:
- Let the number be x.
- Equation: 6x = 48.
- Solve for x: x = 48/6 = 8.
Answer: The number is 8.
Example 3: Solve for x: 5x – 95 = 75.
Solution:
- Add 95 to both sides: 5x = 75 + 95.
- Simplify: 5x = 170.
- Divide by 5: x = 170/5 = 34.
Answer: x = 34.
9. Linear Equation Practice Questions
(Practice questions would be inserted here to reinforce learning, but are not provided in the original article.)
10. FAQs on Linear Equation
What is a Linear Equation? Explain with an Example.
A linear equation is an equation where the highest power of the variable is 1. Its graph always results in a straight line. Examples include 5x + 6 = 1, 42x + 32y = 60, and 7x = 84.
What is the Formula for a Linear Equation?
The formula can be expressed in standard form (Ax + By = C), slope-intercept form (y = mx + c), or point-slope form (y – y₁ = m(x – x₁)). Each form serves a different purpose in solving or graphing linear equations.
Why is a Linear Equation Called Linear?
It’s called linear because its graph is a straight line. The term ‘linear’ directly relates to the line formed when the equation is plotted on a graph.
How do you Solve Linear Equations?
Isolate the variable on one side by performing the same operations on both sides. For example, to solve x – 1 = 5 – 2x, move terms to get 3x = 6, then x = 2.
Can Linear Equations have Fractions?
Yes, linear equations can have fractions, as long as the denominator is a constant. The variables cannot be part of the denominator.
What are Linear Equations in One Variable?
These equations have only one variable and are in the form Ax + B = 0. For example, 9x + 78 = 18 is a linear equation in one variable.
How do You Convert a Linear Equation to Standard Form?
Move all variables to one side and constants to the other, arranging terms so variables are on the left and constants on the right.
What are Linear Equations in two Variables?
These are in the form Ax + By + C = 0, where x and y are variables. An example is 7x + 9y + 4 = 0.
How are Quadratic Equations Different from Linear Equations?
Linear equations have variables with a maximum power of 1, while quadratic equations have at least one term with a variable raised to the second power. Linear equations graph as straight lines, whereas quadratic equations graph as parabolas.
How to Graph Linear Equations?
Convert the equation to y = mx + b form, find at least two points (x, y) that satisfy the equation, plot these points on the graph, and draw a straight line through them.
How to Solve Linear Equations with Fractions?
Solve them the same way as usual equations. Bring variables to one side and constants to the other, then solve for the variable. For example, to solve (2a/3) – 10 = 12, isolate ‘a’ to find a = 33.
Do you have more questions or need further clarification on linear equations? Visit WHAT.EDU.VN to ask your questions and get free, expert answers! Our platform is designed to provide quick, accurate, and helpful information on all your academic inquiries.
Are you finding it difficult to get quick and free answers to your questions? Do you feel lost and unsure where to seek reliable information? Are you worried about the cost of getting expert advice? At WHAT.EDU.VN, we understand these challenges.
We offer a free platform where you can ask any question and receive prompt, accurate answers. Our service connects you with knowledgeable individuals who can provide clear, easy-to-understand explanations. Whether you’re a student, a professional, or simply curious, WHAT.EDU.VN is here to help.
Don’t hesitate—visit WHAT.EDU.VN today and ask your question for free!
Contact Us:
- Address: 888 Question City Plaza, Seattle, WA 98101, United States
- WhatsApp: +1 (206) 555-7890
- Website: what.edu.vn
We’re here to make learning easy and accessible for everyone.